WARNING - the ideas are right, the maths may not be!
1. A planet orbits around a 5
![]() star at a distance of 5 AU's. What is it's period?
star at a distance of 5 AU's. What is it's period?
Let anything underscore 1 relate to the Earth-Sun system.
M2 = 5
![]() = 5M1. a2 = 5AU = 5a1 so
= 5M1. a2 = 5AU = 5a1 so
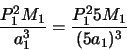 |
(1) |
2. A planet orbits a star in a Period of 3 years at a distance of 2 AU's. What is the star's mass?
Let anything underscore 1 relate to the Earth-Sun system.
P2 = 3 years = 3P1, a2 = 2AU = 2a1 so
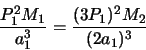 |
(2) |
3. A satellite orbits an 'earth' at a distance of 1000000 km in 30 hours. If we double the distance, what is the new period?
Using the same equations but this time need to set up what subscript 1 and 2 things are. Told in question that satellite orbits at 1000000 km in 30 hours. Therefore a1 = 1000000 km, P1 = 30 hours, M1 unknown. Now second scenario a2 = 2a1 (told in question), want to find P2 and M2 = M1 (same earth type object).
so as before
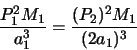 |
(3) |
4. A satellite orbits a planet at a distance of 1000000 km in 30 hours. If we double the mass of the planet and keep the period the same as in (3), what distance does the satellite orbit at?
Now M2 = 2M1 and P2 = 8P1 (found from above) find a2 as before
 |
(4) |
5. (Harder) What is the value of the acceleration produced by a planet that is twice as massive and twice as large as Earth (hint F = m1a = G(m1m2/r2) remembering that the force is felt by you and relate the value of a to the value of g). What would you weight on this planet (assuming mass = 100kg).
So we use two of Newtons laws here
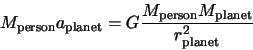 |
(5) |
Now rearrange to have a constant value of one side
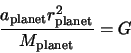 |
(6) |
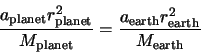 |
(7) |
| (8) |
| (9) |
What would you weight? F=ma = 100 4.9 = 490 N
6. (Sneaky one) Mercury orbits the sun with semi major axis 0.4 AU in 0.25 years. If the mass of Mercury was doubled, what would the period be if the distance was kept at 0.4 AU (Think of the assumptions we make).
Answer is the same. We make the assumption that the mass of mercury is tiny compared to the mass of the Sun so doubling the mass of mercury would NOT effect the calculation.
7. (Using small angle formula but same proportionality idea). At a distance of 12,000km, a planet appears to have an angular size of 4.4''. If the object is moved to 24,000km, what would the angular size be? Do this without calculating the linear size D by treating D*206265 as a constant (which it is the planet is unchanged.)
| (10) |
| (11) |
8. A planet is orbiting a star with semi-major axis a. Does it have a larger period if we double the semi-major axis or half the semi-major axis?
Doubling a) a2 = 2a1 and M2 = M1 so using
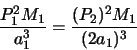 |
(12) |
If we half a) then a2 = 1/2 a1 and then P2 = 1/![]() P1
P1
so Period is larger if we double radius rather than half it (and by thinking about P2 = a3 we could have got to this conclusion).